Have you ever thought about how engineers figure out the height of tall buildings? Or how they know the distance to a mountain? They use angles of elevation and depression. These angles help in many fields like surveying, navigation, and building. We will learn about these angles and see how they are used in real life.
These ideas are more than just theories; they are used every day. For instance, if the angle of elevation from one point to another is 35 degrees, this can help figure out distances or heights. Like how far a tree is from someone watching it, as seen in Example 1. This piece aims to change how we see these angles. It shows us how to solve real-world problems. To learn more, check out this resource on angles of elevation.
Key Takeaways
- The angle of elevation measures how high something looks to the observer.
- The angle of depression is how steeply you must look down to see something.
- These angles help in many areas like surveying and designing buildings.
- They are used to calculate distances, heights, and other angles in navigation.
- Knowing about these angles can improve how we solve problems with elevations and depressions.
Introduction to Angles of Elevation and Depression
Angles of elevation and depression are key in math, especially with trigonometric functions. They form between a horizontal line and the line of sight to an object. This can be above (elevation) or below (depression) the line. Understanding these concepts helps us grasp math ideas and their real-life uses.
For example, the angle of depression to a boat from a cliff is about 35°. Meanwhile, the angle of elevation to a flag marker from the boat is roughly 18°. These measurements are vital in fields like navigation and architecture. Trigonometric functions help us find distances between objects. For instance, using tangent functions, the distance from the boat to the cliff base was found to be 34.3 meters.
Learning to calculate these angles is crucial for solving different problems. Take an observer 20 meters from a building, measuring an angle of elevation at 30°. They can find the building’s height to be about 11.55 meters.
Teachers also show students how to use sine, cosine, and tangent functions. These tools make it easier to figure out unknown distances and heights. They also open doors to understanding complex math concepts. For more information, check out this resource on angles of elevation and.
Defining Angle of Elevation
The angle of elevation is essential in how we see objects above us. It’s the angle between a horizontal line from our eye and the line of sight aiming up at an object. How high the object is affects this angle, highlighting its importance in trigonometry.
What is an Angle of Elevation?
An angle of elevation is measured from a horizontal line to an object above. This idea is practical, especially in calculating distances and heights. Trigonometry uses functions like the tangent to measure these accurately. For instance:
Tan θ = Opposite Side / Adjacent Side
In simple terms, the opposite side is the object’s height, and the adjacent side is the distance from the observer to the object. This helps in determining the object’s height accurately when we know the distance and angle.
Real-life Examples of Angle of Elevation
The angle of elevation is used in many areas like architecture, aviation, and surveying. For example, if someone stands 100 meters from a tower and sees it at a 60-degree angle, trigonometry shows the tower’s height to be around 173.20 meters. This shows the role of angles in everyday life and how trigonometry solves real problems.
Consider a pilot flying towards a tower from 5,000 feet up at a 10-degree angle. Calculating the distance to the tower ensures a safe approach. This highlights how vital angle of elevation is in making plans and decisions.
Understanding Angle of Depression
The angle of depression is key in several areas. It helps measure angles from a horizontal line down to a point below. This angle, measured in degrees, is crucial in daily tasks like navigation and engineering.
What is an Angle of Depression?
An angle of depression happens between a horizontal line and a line of sight to a lower point. It’s usually seen from eye level to something below the observer. This measure is vital for finding distances and heights in real life.
Examples of Angle of Depression in Daily Life
Knowing about the angle of depression applies in many ways, for example:
- Aviation: Pilots need this angle to land safely. They adjust their path using this angle.
- Surveying: Surveyors find out how deep things like wells are, using math.
- Architecture: Architects use these angles for designing good-looking buildings.
In these situations, being accurate with trigonometric measurements is very important. By using inverse tangent, professionals can figure out the needed angle of depression. This shows how trigonometry and real-world tasks use these angles together.

The Relationship Between Angles of Elevation and Depression
The angles of elevation and depression share an interesting symmetry. It helps us understand geometry better. When you look at two objects along a horizontal line, the angle you look up at is equal to the angle you look down from the other side. This idea comes from how parallel lines work and something called alternate interior angles.
Using this fact makes calculating height and distance easier. It’s really useful in navigation and surveying. For example, if a surveyor wants to know how tall a building is, they measure the angle of elevation from a certain distance. Then, they use trigonometry to find the answer. This shows how valuable knowing about elevation and depression angles can be.
These two angles have a deep connection in geometry. Knowing how they work together makes solving problems easier. It helps get accurate measurements in many fields. For more information, check out this resource. It gives more insight into angles of elevation and depression and trigonometry.
| Angle Type | Definition | Application |
|---|---|---|
| Angle of Elevation | The angle formed between the horizontal line and a line of sight up to an object. | Used in calculating heights of structures. |
| Angle of Depression | The angle formed between the horizontal line and a line of sight down to an object. | Applied in navigation and determining depths. |
Trigonometric Functions: A Key to Understanding Angles
Trigonometric functions help us calculate angles for elevation and depression. They let us find the height and distance we need in different situations. The way these angles link to right triangles is key for precise calculations.
How Trigonometry Relates to Elevation and Depression
When we look up at things like buildings or trees, we’re seeing angles of elevation. Meanwhile, angles of depression happen when we look down at something on the ground. Trigonometry allows us to form right triangles. This makes it easier to work out an object’s height or how far away it is.
These methods are used in many real-world cases. They help us figure out how tall a lighthouse is from a boat or the distance to a plane from radar stations. In these examples, knowing about angles and trigonometry is very important.
Using Tangent to Calculate Angles
The tangent ratio is essential for working out elevation and depression. It shows the connection between an angle of elevation and a right triangle’s sides. In essence, the tangent of an angle compares the object’s height to the distance from it:
Tangent (θ) = Opposite (height) / Adjacent (distance)
This ratio lets us find unknown lengths or heights by using the angle of elevation. If we know a building’s top angle is 30 degrees and it’s 50 feet away, we can calculate its height with the tangent function.
| Angle (Degrees) | Distance (Feet) | Height (Feet) | Tangent Ratio |
|---|---|---|---|
| 30 | 50 | 25 | 0.577 |
| 45 | 50 | 50 | 1 |
| 60 | 50 | 86.6 | 1.732 |
Architects, engineers, and navigators use these ideas to solve problems with angles. They can handle elevation and depression issues smoothly.
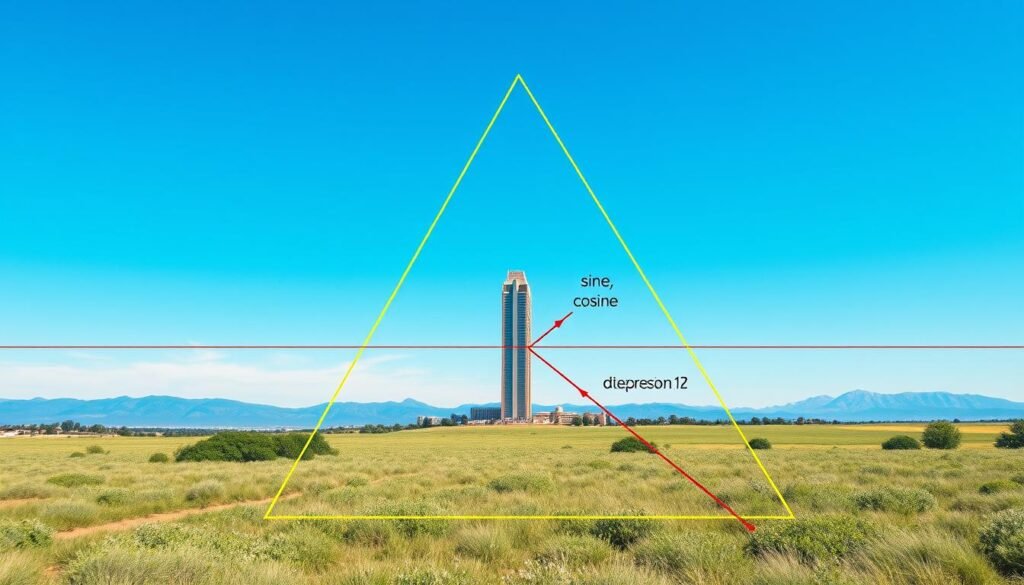
Applications of Angles in Different Fields
Angles are very important in many areas, like surveying, civil engineering, and architecture. People working in these areas depend on accurate angle measurements. This ensures their projects are safe and successful.
Importance of Angles in Surveying
Knowing about angles of elevation and depression is key in surveying. These angles help measure land and find out how high things are. With trigonometry, surveyors measure distances between places. This is crucial for making maps and setting boundaries.
The method helps to accurately find a building’s height or how far away an aircraft is from a radar station.
Applications in Civil Engineering and Architecture
Angles are very necessary in civil engineering and architecture. They help design buildings, bridges, and roofs. Architectural designs often use right triangles for strength and support.
Engineers use angles to figure out the forces in structures to keep them safe. Also, the angle of elevation is used to determine the right height for buildings. This makes sure the buildings are both useful and look good.
There are many real-life examples. These include designing ramps for better access and sloping roofs for drainage. Knowing about angles makes designing easier. It also helps make buildings safe and efficient.
Using Angle of Elevation in Navigation
The angle of elevation is crucial for navigators, especially mariners and aviators. It helps them know where they are compared to landmarks or high points. For sailors, knowing the angle of elevation helps measure how far they are from a harbor. This is key for safe and accurate course plotting.
For pilots, the angle of elevation is just as important. They use math to figure out how high their plane is above the ground. Correct measurements help avoid mistakes in altitude and keep planes safe when flying close to the ground or landing.
Understanding this angle also matters for surveyors and architects. It helps them be precise in their work. They use accurate angle measuring to improve safety and efficiency in construction. Knowing about elevation angles helps in many professions.
Angle Measurements and Their Importance
Understanding how to measure angles is key in fields like trigonometry, surveying, and navigation. It’s important to know about degrees, minutes, and seconds. These units help us be very precise. For example, degrees are the main way to measure angles. Minutes and seconds let us be even more exact in our measurements.
This is useful in real situations. Like when a pilot needs to know how to tilt towards the ground crew. They use angles, for instance around 12.25°, to communicate clearly and correctly.
Understanding Degrees, Minutes, and Seconds
Being exact with angles matters a lot, not just in theory but in real life too. Take measuring the height of something when you know the angle is 21.8°. It shows why understanding angular units is practical. Knowing how to switch between degrees and minutes or seconds is key.
This skill helps in measuring distances or heights accurately. It ensures our results are right on target.
Measuring Angles Accurately
Getting angles right is essential for navigation and engineering. By using trigonometric ratios like sine, cosine, and tangent, we can work out angles well. Consider understanding a 45° angle of depression at the Grand Canyon. These calculations can be crucial for designing buildings or flying a plane.
With tools like guides on coping with complex scenarios, learning these concepts becomes easier. They show the importance of accurate measurements for success in many areas.